Beyond the Basics: Uncover the Surprising Truth About Rational Numbers

Here is a persuasive advertisement for ghostwriting services for the discipline of Mathematics, specifically focused on Rational Numbers:

**Unlock Top-Grade Math Papers with Our Expert Ghostwriting Services**

Struggling to grasp the concept of rational numbers? Do you find it challenging to perform operations with multiple representatives? Our team of expert math ghostwriters is here to help!

**Our Services:**

* Customized math papers on rational numbers, tailored to your specific needs and requirements
* Expertly crafted solutions to complex math problems, ensuring accuracy and precision
* Comprehensive support for assignments, essays, and projects, covering topics such as:
+ Definition of rational numbers as a set of equivalent fractions
+ Operations with multiple representatives, including addition and more
+ Real-world applications of rational numbers

**Why Choose Our Ghostwriting Services?**

* **Expertise**: Our team of math experts has a deep understanding of rational numbers and related concepts, ensuring that your paper is accurate and informative.
* **Time-Saving**: Let us handle the research and writing, so you can focus on other important aspects of your academic life.
* **Confidentiality**: Our services are completely confidential, ensuring that your paper is kept private and secure.
* **High-Quality**: We guarantee top-grade papers that meet your professor’s expectations, every time.

**Get Started Today!**

Don’t let math struggles hold you back. Our ghostwriting services are designed to help you succeed. Contact us now to learn more about how we can assist you with your rational numbers paper.

Order now and receive a well-researched, expertly written paper that will impress your professors and help you achieve academic success!

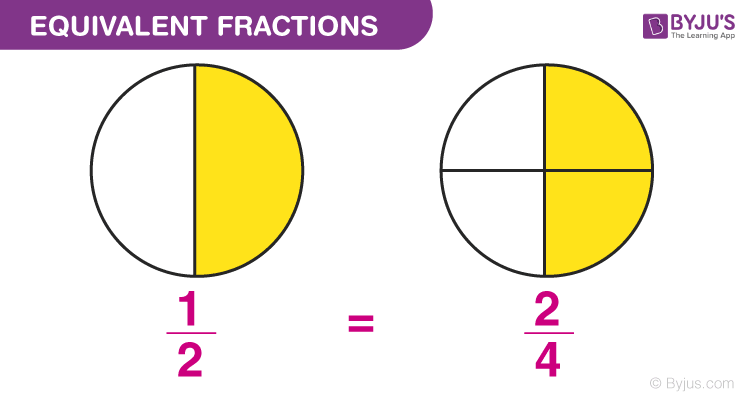
The rational numbers are the first example we usually encounter of an quotient set. For example, “\(\frac{1}{2}\)” and “\(\frac{2}{4}\)” are different literal fractions – they have different numerators and different denominators, so they are not identical – but they represent the same ratio. A rational number is not a single fraction, but an infinite set of fractions each of which can serve as a representative of its set. Indeed, to add fractions it is necessary to select representatives with the same denominator.

Negative numbers are often initially viewed with suspicion – after all, how can anything be less than zero? Of course, in the modern world, all it takes is having a credit card to know that negative numbers are perfectly real. We can also think of negative numbers as telling us direction – a speed of \(-5\) miles per hour indicates that the car is moving at \(5\,\)mph in reverse.

The standard symbols for the sets of integers and rational numbers are \(\mathbb{Z}\) and \(\mathbb{Q}\). The “\(\mathbb{Q}\)” stands for “quotient,” but the choice of “\(\mathbb{Z}\)” for “integers” seems strange – should not it be “\(\mathbb{I}\)” for “integer?” In fact, the symbol _is_ chosen for the first letter of the word for integers; it is just that it was named in German, with “\(\mathbb{Z}\)” short for _Zahlen_, the German word for “integer.” The rational numbers contain the integers as a subset which in turn contain the natural numbers as a subset. It is natural to ask the question what else do we know for \(\mathbb{Z}\)? We have inequalities. The sign “\(<\)" is a relation on \(\mathbb{Z}\) that is subject to the following _order properties_:

* If \(a,b\in\mathbb{Z}\), then one and only one of the following holds: \(a

We adopt the standard notation. That is, if \(aa\) and say \(b\) is greater than \(a\). The notation “\(\geq\)” is defined analogously.

### Fields

The rational numbers are our first example of an important mathematical structure known as a _field_. A field is a set \(\mathbb{F}\) with addition and multiplication operations such that

* Both operations are associative and commutative.
* The operations interact via the _distributive law_ \[a(b+c)=ab+ac\quad\text{and}\quad(a+b)c=ac+bc.\]
* There are additive and multiplicative identity elements (i.e., \(0\) and \(1\)).
* Every element \(x\in\mathbb{F}\) has an additive inverse \(-x\in\mathbb{F}\) and every nonzero element \(x\in\mathbb{F}\) has a multiplicative inverse \(x^{-1}\in\mathbb{F}\).

We can use the field properties to prove theorems that hold for all fields. For example, see the following theorem:

**Theorem 3** (Additive Cancellation Law).: _If \(\mathbb{F}\) is a field and \(\alpha,\beta,\gamma\in\mathbb{F}\) satisfy \(\alpha+\beta=\alpha+\gamma\), then \(\beta=\gamma\)._

Proof

: Consider \(-\alpha+(\alpha+\beta)\). Since \(\alpha+\beta=\alpha+\gamma\), we have

\[-\alpha+(\alpha+\beta)=-\alpha+(\alpha+\gamma).\]

Now, the fact that the addition is associative says

\[(-\alpha+\alpha)+\beta=(-\alpha+\alpha)+\gamma,\]

and we then have

\[0+\beta=0+\gamma.\]

This says

\[\beta=\gamma\]

as required.

Once we have proved a result, we can use it to prove other results. For example, see the following theorem:

Proof

: First, we note that \(0=0+0\). Then

\[0\alpha=(0+0)\alpha=0\alpha+0\alpha\]

by the distributive law; on the other hand

\[0\alpha=0\alpha+0=0\alpha+0\alpha.\]

Then by additive cancellation we have \(0=0\alpha\).

**Theorem 5**.: _If \(\mathbb{F}\) is a field in which \(0\) has a multiplicative inverse, then every element of \(\mathbb{F}\) equals zero._

Proof

: Let \(\mathbb{F}\) be a field in which \(0\) has a multiplicative inverse \(0^{-1}\). Then for any \(\alpha\in\mathbb{F}\), we have

\[\alpha=1\alpha=(00^{-1})\alpha=0(0^{-1}\alpha)=0.\qed\]

Note that this explains why we cannot just declare \(\infty=\frac{1}{0}\) and make math much simpler – any field in which zero has a multiplicative inverse contains only one element, namely zero. Thus, we have to either give up the field axioms, give up on zero having a multiplicative inverse, or settle for a number system with only one number.

### 1.3 Modular Arithmetic

Another very useful number system (a well, infinite set of number systems) which is unfamiliar to most outside of mathematics and computer science is the _integers modulo_\(n\). Let \(n\) be a positive integer. Then the set of integers modulo \(n\) is the set

\[\mathbb{Z}_{n}=\{0,1,2,\ldots,n-1\}\]

of remainders after long division by \(n\). An element of \(\mathbb{Z}_{n}\) is an equivalence class, like rational numbers, except that where two fractions represent the same rational number if one can be obtained from the other by multiplying top and bottom by the same integer, and two integers represent the same element of \(\mathbb{Z}_{n}\) if one can be obtained from the other by adding a multiple of \(n\).

In particular, we can do arithmetic mod \(n\) by doing ordinary arithmetic with the extra rule that whenever our numbers get outside the range \(\{0,1,2,\ldots,n-1\}\), we divide by \(n\) and keep only the remainder, i.e., add or subtract \(n\) repeatedly until we are back in \(\{0,1,2,\ldots,n-1\}\). Where \(\mathbb{Z}\) forms a number line, \(\mathbb{Z}_{n}\) forms a number circle, like an analog clock – in fact, we use mod \(12\) (and mod \(60\)) arithmetic for telling time (Figure 1.11).

**Example 15**.: In \(\mathbb{Z}_{4}\), we have

\[2(3+3)+3(2-3)=2(6)+3(-1)=2(2)+3(3)=4+9=0+1=1.\]

**Example 16**.: We can do reduction mod \(n\) at every step, or we can save it all up for the last step, and we will get the same result. For example, in \(\mathbb{Z}_{5}\), \((2+4)3-4(3+3)=2\):

\[\begin{array}{rllllll}(2+4)3-4(3+3)&=&(6)3-4(6)&&\\ &=&(1)3-4(1)&&\\ &=&3-4&\text{or}&\\ &=&-1&\\ &=&4&\end{array}\quad\text{or}\quad\quad\begin{array}{rllll}(2+4)3-4(3+3)&=&1 8-24\\ &=&-6\\ &=&-1\\ &=&4.\end{array}\]

Modular arithmetic differs from ordinary arithmetic in some important ways.

* A _zero divisor_\(x\neq 0\) has the property that \(xy=0\) for some \(y\neq 0\). In integer arithmetic, there are no zero divisors, but there can be zero divisors in \(\mathbb{Z}_{n}\) depending on \(n\). For instance, in \(\mathbb{Z}_{4}\), \(2\) is a zero divisor since \(2(2)=4=0\).
* An element \(x\in\mathbb{Z}_{n}\) is a _unit_ if it has a multiplicative inverse, i.e., if there is an \(x^{-1}\in\mathbb{Z}_{n}\) such that \(xx^{-1}=1\). For example, in \(\mathbb{Z}_{7}\), we have \[\begin{array}{r|l}x&x^{-1}\\ \hline 1&1\\ 2&4\\ 3&5\\ 4&2\\ 5&3\\ 6&6\end{array}\] since \(2(4)=8=1\), \(3(5)=15=1\), and \(6(6)=36=1\) mod \(7\). In a field, every nonzero element must be a unit.

We have seen that \(\mathbb{Q},\mathbb{R}\), and \(\mathbb{C}\) are fields, while \(\mathbb{N}\) and \(\mathbb{Z}\) are not. \(\mathbb{N}\) lacks additive and multiplicative inverses for elements greater than \(1\), and while

Figure 11: Integers and integers modulo \(n\)includes additive inverses for everything, the only elements in \(\mathbb{Z}\) with multiplicative inverses are \(1\) and \(-1\). We say \(\mathbb{Z}\) is an _integral domain_, a type of number system which does not contain zero divisors.

**Example 17**.: Note that \(\mathbb{Z}_{5}\) is a field. To prove this, one needs to verify that all of the field axioms are satisfied. Most of these are straightforward: Multiplication and addition are associative, commutative, and distributive in \(\mathbb{Z}_{5}\) because they are associative, commutative, and distributive in \(\mathbb{Z}\) before reducing mod \(5\), and reducing equal quantities mod \(5\) yields equal quantities in \(\mathbb{Z}_{5}\). The interesting part is to check the additive and multiplicative inverses, which we can do by brute force since \(\mathbb{Z}_{5}\) has only five elements.

\begin{tabular}{|c|c|c|c|c|} \hline \(\alpha\) & \(-\alpha\) & & \(\alpha^{-1}\) & \\ \hline \hline
0 & 0 & \(0+0=0\) & \(-\) & \\
1 & 4 & \(1+4=5=0\) & 1 & \(1(1)=1\) \\
2 & 3 & \(2+3=5=0\) & 3 & \(2(3)=6=1\) \\
3 & 2 & \(3+2=5=0\) & 2 & \(3(2)=6=1\) \\
4 & 1 & \(4+1=5=0\) & 4 & \(4(4)=16=1\) \\ \hline \end{tabular}

Note that \(\mathbb{Z}_{n}\) may or may not be a field depending on the value of \(n\); see the exercises for more.

### Exercises

1. Prove that in every field, we have the _multiplicative cancellation property_, i.e., that \(\alpha\beta=\alpha\gamma\) implies \(\beta=\gamma\).

2. Recall that a nonzero element of a number system \(\beta\neq 0\) is a _zero divisor_ if there is a nonzero element \(\alpha\neq 0\) such that \(\alpha\beta=0\) and that \(\beta\) is a _unit_ if there exists an element \(\beta^{-1}\) such that \(\beta\beta^{-1}=1\). Prove that no element can be both a unit and a zero divisor.

3. Make the addition and multiplication tables for the field \(\mathbb{Z}_{7}\).

4. Identify the units of \(\mathbb{Z}_{10}\) by finding their multiplicative inverses and the zero divisors.